Twisted beam
1 Model
For this test, a beam with a solid rectangular cross section is fixed on one end. The cross section is twisted from 0 to 90 degrees along the beam, as shown in the image below.
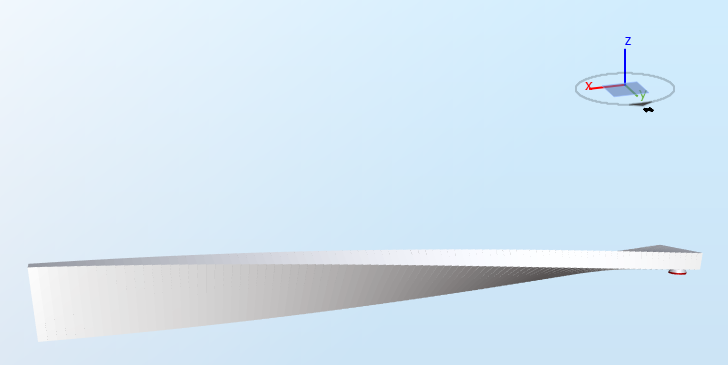
The length of the beam is
$$L = 12\text{ m}$$
, the height and width of the cross section are $$h = 0.32\text{ m}$$
and $$w = 1.1\text{ m}$$
, respectively. the Elastic modulus is $$E=29.0\cdot10^6\text{ Pa}$$
and the Poisson ratio is $$\nu = 0.22$$
. More details can be found in Macneal et al. (1985). In Ashes, the model is divided into 91 elements.2 Benchmarks
2.1 In-plane shear
For this benchmark, a force
$$F_z = -1\text{ N}$$
is applied in the z-direction, as shown in the figure below.
According to Macneal et al. (1985), the expected displacement of the tip of the beam is
$$d_z = -0.005424\text{ m}$$
.2.2 Out-of-plane shear
For this benchmark, a force
$$F_y = 1\text{ N}$$
is applied in the y-direction, as shown in the figure below.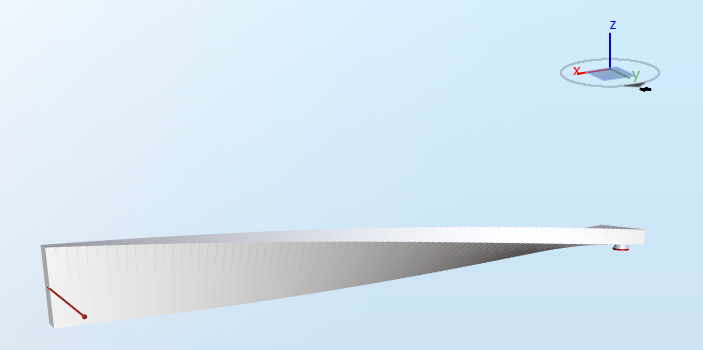
According to Macneal et al. (1985), the expected displacement of the tip of the beam is
$$d_y = 0.001754\text{ m}$$
3 Results
The tests are considered passed when the last 20% of the time series from Ashes is within 1% of the results from Macneal et al. (1985).
The report for this test can be found on the following link: