Timoshenko blade
This test uses the same benchmark as the Cantilever beam based on the book from Bell (1987b), but a blade is used instead of a support section.
1 Benchmark
The table above Figure 6.6 in Bell (1987b) analyses the tip displacement of a cantilever beam subjected to a point load at its tip. The beam has an HE300B cross section, with a given shear area
$$A_S = 0.029\text{ m}^2$$
, a second moment of inertia $$I = 0.2517\text{ m}^4$$
, an Elastic modulus $$E = 210\cdot10^9\text{ Pa}$$
and a Poisson ratio of $$\nu = 0.3$$
. The height of the cross section is $$h = 0.3\text{ m}$$
.For different ratios of length
$$l$$
over height $$h$$
of the beam, the table gives the expected proportion of displacement with and without considering Shear deformation, which in Ashes can be modelled by Timoshenko and Euler-Bernoulli elements, respectively.The data from the table is reproduced below.
$$l/h$$ | 2 | 5 | 10 | 20 |
$$\sigma$$ | 2.88 | 1.30 | 1.08 | 1.02 |
where
$$\sigma$$
is the ratio of the displacement witth Timoshenko elements over the displacement with Euler-Bernoulli elements.For this test, we create a blade with the same structural characteristics as those listed above.
We remove gravity and aerodynamic loads and we apply a load
$$P = 100\text{ kN}$$
at the tip of the blade, as shown in the figure below: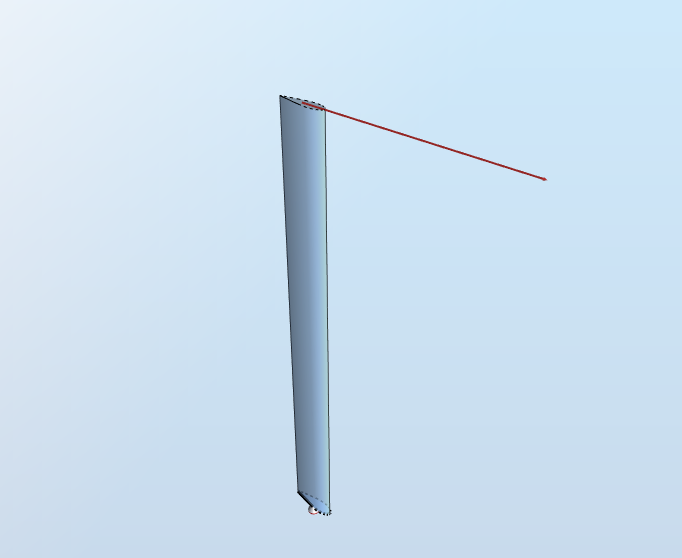
The analytical solution of the tip displacement for an Euler-Bernoulli beam can be found for example in Wikipedia as
$$w_E = \frac{Pl^3}{3EI}$$
By using the characteristics of the cross-section and the Euler-Bernoulli analytical solution, we can find the expected tip displacements for different blade lengths and beam theories, as summarised in the table below:
$$l\text{ }(m)$$ | 0.6 | 1.5 | 3 | 6 |
$$w_E\text{ }(m)$$ | $$1.36\cdot10^{-4}$$ | $$0.00213$$ | $$0.0170$$ | $$0.136$$ |
$$w_T\text{ }(m)$$ | $$3.92\cdot10^{-4}$$ | $$0.00277$$ | $$0.0184$$ | $$0.139$$ |
2 Results
The test is considered passed if the results from Ashes lie within 0.5% of the Benchmark values
The report for this test can be found on the following link: